Some topics developed at SiMMaS

Skyrmions
Transport of skyrmions on racetracks. Analytical treatment with Thiele’s model

Magnetic skyrmions can be considered as magnetic bits due to their nanometric size and topologically protected stability. We have studied the dynamics of such skyrmions in racetrack geometries and, in general, in confined geometries. Analytical expressions for the trajectories of skyrmions in confined geometries (including long tracks and squared samples) when driven by polarized electric currents. The force exerted by the borders is derived as a function of the material parameters (anisotropy, Dzyaloshinskii-Moriya, and exchange constants) and incorporated in the equation of motion of the skyrmions.

Trajectories for a skyrmion with squared borders, for the case of ac driving current of different values of the frequency. A ressonance effect is seen in the third picture. The value of the driving current is the same in all cases

More information: https://doi.org/10.1103/PhysRevB.94.184104

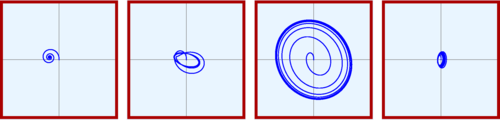
Transport of skyrmions on realistic racetracks. Granularity and temperature

Skyrmions, as as bits of information, that can be transported along nanoracetracks. However, temperature, defects, and/or granularity can produce diffusion, pinning, etc. These effects may cause undesired errors in information transport may be compromising the feasibility of the device. We have performed simulations of a realistic system where both the (room) temperature and sample granularity are taken into account. Key feasibility magnitudes, such as the success probability of a skyrmion traveling a given distance along the racetrack, are calculated. The model proposed is based on the Fokker–Planck equation resulting from Thiele’s rigid model for skyrmions.

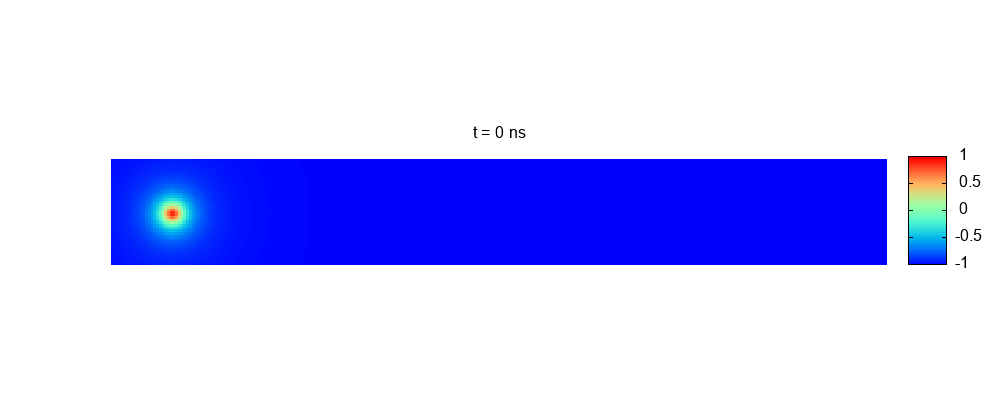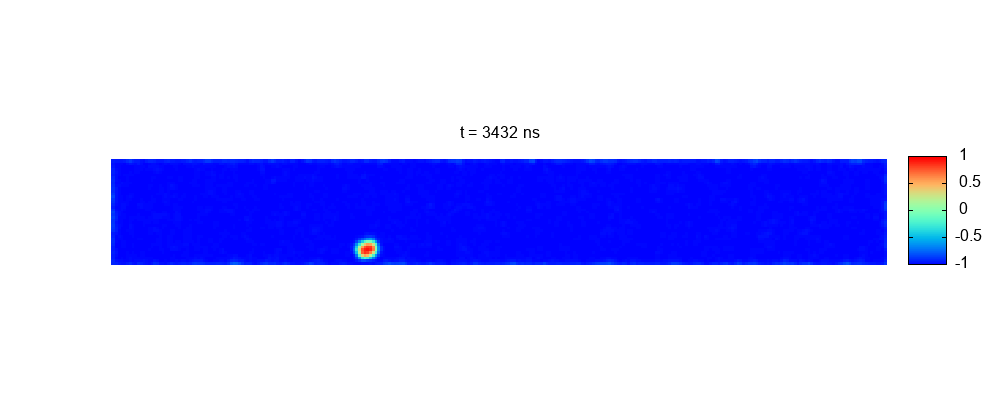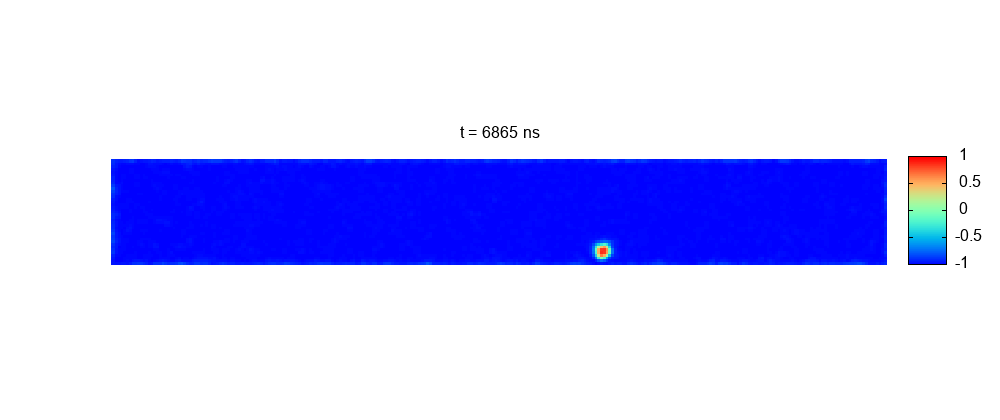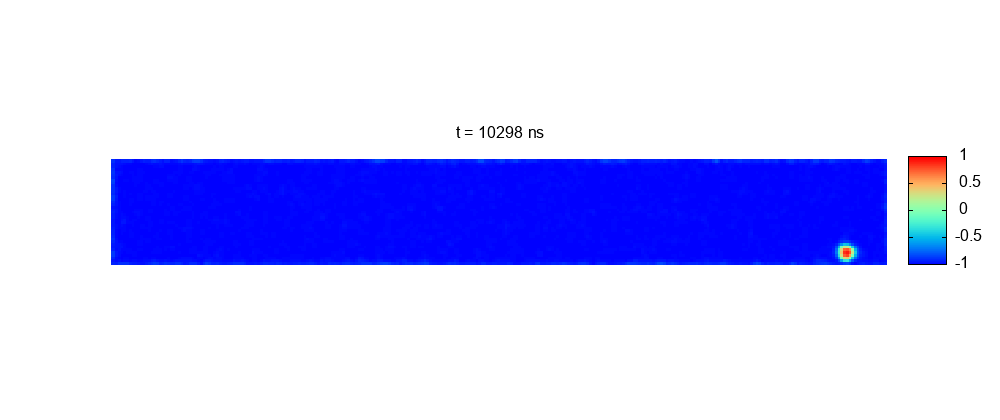
More information: https://pubs.rsc.org/en/content/articlelanding/2023/na/d3na00464c

Generation of skyrmions by template imprinting

Magnetic skyrmions are promising candidates as information carriers in new generation magnetic memories. The physical conditions for nucleating and stabilizing skyrmions depend largely on many parameters such as temperature or the type of materials. We have demonstrate how skyrmions can be imprinted in ultrathin ferromagnetic films, either individually and also in large numbers by bringing a magnetic nanostructured template close to the film.

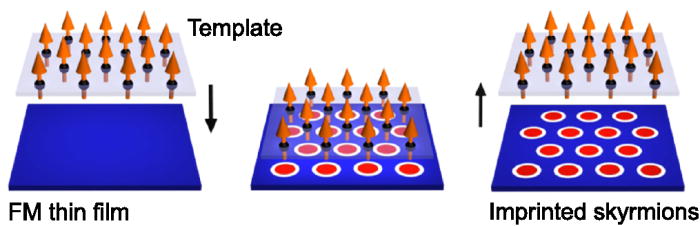
Sketch of the proposed procedure for imprinting skyrmions. A magnetic template is approached to an initially ferromagnetic (FM) thin film (left); under the appropriate conditions, a lattice of skyrmions is nucleated (center) and stabilized when the template, which remains unaffected, is removed away (right).

More Information: https://doi-org.are.uab.cat/10.1063/1.4932090

Hybrid Systems FM-SC
Magnetic multistates

We have developed simulations fro accounting for the interaction of a FM disk and a superconducting disk. The interaction is accounted for by solving simultaneously the Brown and London equations. We have discussed the effect of the different parameters on the results and some possible applications such as multivalued logic, storage, and skyrmionic metamaterials.

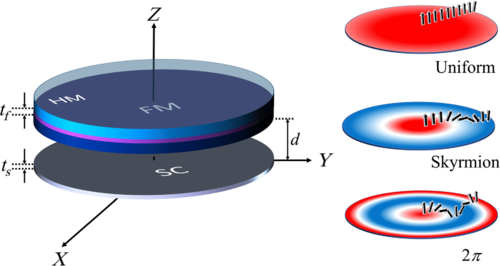
Sketch of the studied sytem and the possible magnetic structures present in the ferromagnetic layer. During a hysteresis loop, the magnetic state of the ferromagnet can change from one state to the other, being hihgly influcence by the presence of the superconducting layer.

More information and details: 10.1103/PhysRevApplied.17.034069

Short questions/answers about this topics

What is a skyrmion?

A magnetic skyrmion is a topologically stable, swirling spin texture in a magnetic material. It consists of a localized region where the magnetization vector rotates continuously, forming a vortex-like structure. Skyrmions exhibit unique properties and have potential applications in magnetic storage, spintronics, and information processing.

Which is the Landau-Lifshitz-Gilbert equation?

The Landau-Lifshitz-Gilbert (LLG) equation is a fundamental equation in magnetism that describes the dynamics of a magnetization distribution in a magnetic material. It is given by:

dM/dt = –γ M x Heff + α M x (dM/dt)

M represents the magnetization distribution, t is time, γ is the gyromagnetic ratio, Heff is the effective magnetic field, α is the damping parameter, and dM/dt is the time derivative of the magnetization vector.

The first term on the right-hand side represents the precession of the magnetization around the effective magnetic field, while the second term accounts for the damping effect, which tends to align the magnetization with the effective field.

Page under construction